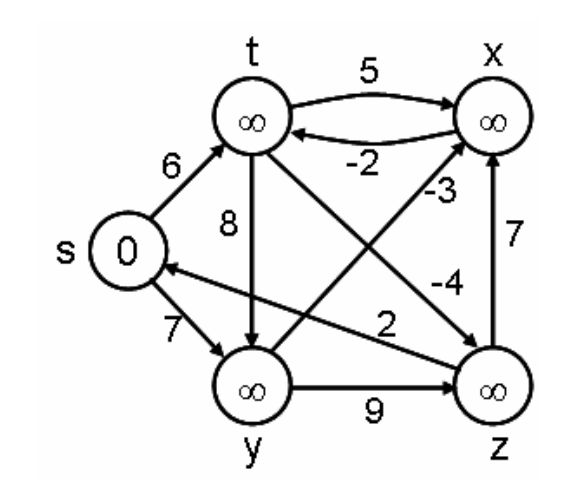
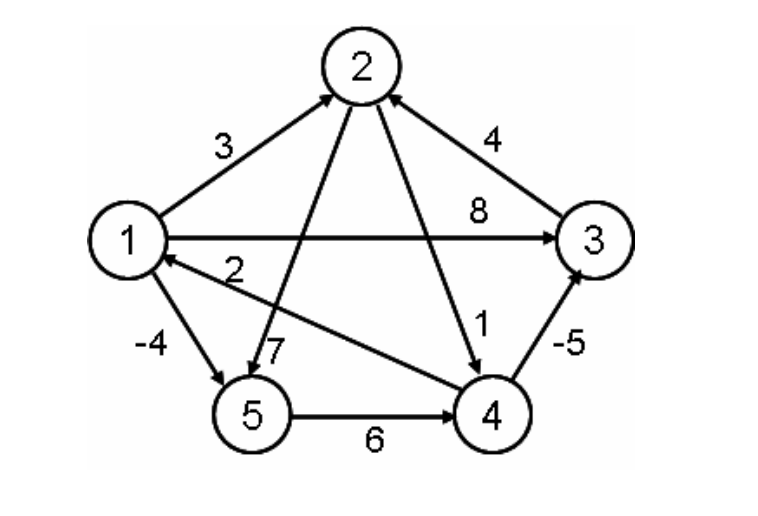
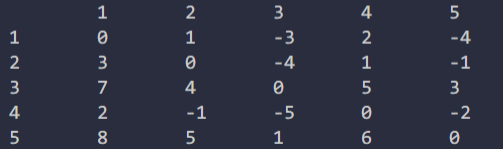
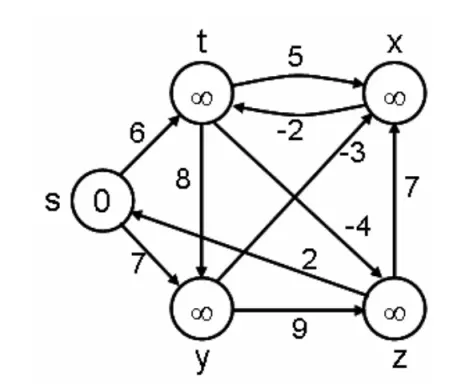
1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
56
57
58
59
60
61
62
63
64
65
66
67
68
69
70
71
72
73
74
75
76
77
78
79
80
81
82
83
84
85
86
87
88
89
90
91
92
93
94
95
96
97
98
99
100
101
102
103
104
105
106
107
108
109
110
111
112
113
114
115
116
117
118
119
120
121
122
123
124
125
126
127
128
129
130
131
132
133
134
135
136
137
138
139
140
141
142
143
144
145
146
147
148
149
150
151
152
153
154
155
156
157
158
159
160
161
162
163
164
165
166
167
168
169
170
171
172
173
174
175
176
177
178
179
180
181
182
183
184
185
186
187
188
189
190
191
192
193
194
195
196
197
198
199
200
201
202
203
204
205
206
207
208
209
210
211
212
213
214
215
216
217
218
219
| import java.util.*;
public class ShortestPath {
static class Vertex {
private final static int infinite_dis = Integer.MAX_VALUE;
private String name;
private boolean known;
private int adjuDist;
private Vertex parent;
public Vertex() {
this.known = false;
this.adjuDist = infinite_dis;
this.parent = null;
}
public Vertex(String name) {
this();
this.name = name;
}
public Vertex getParent() {
return parent;
}
public void setParent(Vertex parent) {
this.parent = parent;
}
public boolean equals(Object obj) {
if (this.getName() == ((Vertex) obj).getName()) {
return true;
}
if (this.name == null) {
throw new NullPointerException("name of Vertex to be compared cannot be null!");
} else {
return false;
}
}
public static int getInfiniteDis() {
return infinite_dis;
}
public String getName() {
return name;
}
public void setName(String name) {
this.name = name;
}
public boolean isKnown() {
return known;
}
public void setKnown(boolean known) {
this.known = known;
}
public int getAdjuDist() {
return adjuDist;
}
public void setAdjuDist(int adjuDist) {
this.adjuDist = adjuDist;
}
}
static class Edge {
private Vertex startVertex;
private Vertex endVertex;
private int weight;
public Edge(Vertex startVertex, Vertex endVertex, int weight) {
this.startVertex = startVertex;
this.endVertex = endVertex;
this.weight = weight;
}
public Vertex getStartVertex() {
return startVertex;
}
public void setStartVertex(Vertex startVertex) {
this.startVertex = startVertex;
}
public Vertex getEndVertex() {
return endVertex;
}
public void setEndVertex(Vertex endVertex) {
this.endVertex = endVertex;
}
public int getWeight() {
return weight;
}
public void setWeight(int weight) {
this.weight = weight;
}
}
private List<Vertex> vertexList;
private Map<Vertex, List<Edge>> ver_edgeList_map;
public ShortestPath(List<Vertex> vertexList, Map<Vertex, List<Edge>> ver_edgeList_map) {
this.vertexList = vertexList;
this.ver_edgeList_map = ver_edgeList_map;
}
public void setRoot(Vertex v) {
v.setParent(null);
v.setAdjuDist(0);
}
private void updateChildren(Vertex v) {
if (v == null) {
return;
}
if (ver_edgeList_map.get(v) == null || ver_edgeList_map.get(v).size() == 0) {
return;
}
List<Vertex> childrenList = new LinkedList<Vertex>();
for (Edge e : ver_edgeList_map.get(v)) {
Vertex childVertex = e.getEndVertex();
if (!childVertex.isKnown()) {
childVertex.setKnown(true);
childVertex.setAdjuDist(v.getAdjuDist() + e.getWeight());
childVertex.setParent(v);
childrenList.add(childVertex);
}
int nowDist = v.getAdjuDist() + e.getWeight();
if (nowDist >= childVertex.getAdjuDist()) {
continue;
} else {
childVertex.setAdjuDist(nowDist);
childVertex.setParent(v);
}
}
for (Vertex vc : childrenList) {
updateChildren(vc);
}
}
public void shortestPathTravasal(int startIndex, int destIndex) {
Vertex start = vertexList.get(startIndex);
Vertex dest = vertexList.get(destIndex);
String path = "[" + dest.getName() + "]";
setRoot(start);
updateChildren(vertexList.get(startIndex));
int shortest_length = dest.getAdjuDist();
while ((dest.getParent() != null) && (!dest.equals(start))) {
path = "[" + dest.getParent().getName() + "] --> " + path;
dest = dest.getParent();
}
System.out.println("[" + vertexList.get(startIndex).getName() + "] to [" + vertexList.get(destIndex).getName()
+ "] ShortestPath shortest path: " + path);
System.out.println("shortest length:" + shortest_length);
}
public static void main(String[] args) {
Vertex s = new Vertex("s");
Vertex t = new Vertex("t");
Vertex x = new Vertex("x");
Vertex y = new Vertex("y");
Vertex z = new Vertex("z");
List<Vertex> verList = new LinkedList<ShortestPath.Vertex>();
verList.add(s);
verList.add(t);
verList.add(x);
verList.add(y);
verList.add(z);
Map<Vertex, List<Edge>> vertex_edgeList_map = new HashMap<Vertex, List<Edge>>();
List<Edge> sList = new LinkedList<ShortestPath.Edge>();
sList.add(new Edge(s, t, 6));
sList.add(new Edge(s, y, 7));
List<Edge> tList = new LinkedList<ShortestPath.Edge>();
tList.add(new Edge(t, y, 8));
tList.add(new Edge(t, x, 5));
List<Edge> xList = new LinkedList<ShortestPath.Edge>();
xList.add(new Edge(x, t, -2));
List<Edge> yList = new LinkedList<ShortestPath.Edge>();
yList.add(new Edge(y, x, -3));
yList.add(new Edge(y, z, 9));
List<Edge> zList = new LinkedList<ShortestPath.Edge>();
zList.add(new Edge(z, x, 7));
vertex_edgeList_map.put(s, sList);
vertex_edgeList_map.put(t, tList);
vertex_edgeList_map.put(x, xList);
vertex_edgeList_map.put(y, yList);
vertex_edgeList_map.put(z, zList);
ShortestPath g = new ShortestPath(verList, vertex_edgeList_map);
g.shortestPathTravasal(0, 1);
g.shortestPathTravasal(0, 2);
g.shortestPathTravasal(0, 3);
g.shortestPathTravasal(0, 4);
}
}
|